The Reverberant Shear Wave Fields

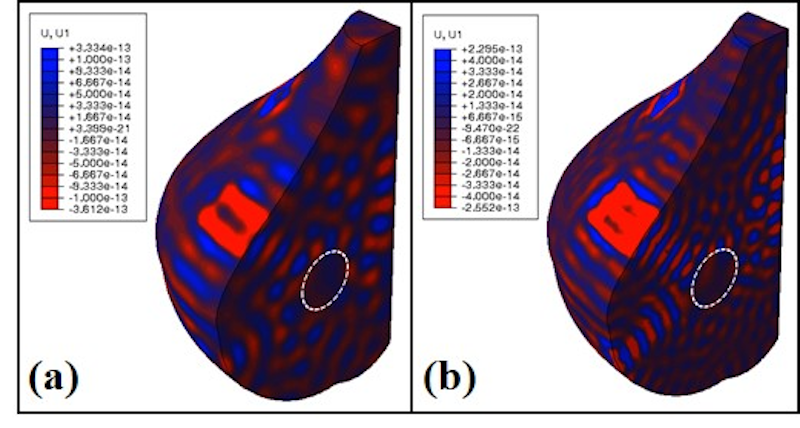
The determination of shear wave speed is an important subject in the field of elastography, since elevated shear wave speeds can be directly linked to increased stiffness of tissues. MRI and ultrasound scanners are frequently used to detect shear waves and a variety of estimators are applied to calculate the underlying shear wave speed. However, multiple reflections from organ boundaries and internal inhomogeneities and mode conversions can create a complicated field in time and space. Thus, we derived the mathematics of multiple component shear wave fields and derive the basic properties from which efficient estimators can be obtained. We approached this problem from the historic perspective of reverberant fields, a conceptual framework used in architectural acoustics and related fields. The framework can be recast for the alternative case of shear waves in a bounded elastic media, and the expected value of displacement patterns in shear reverberant fields have been derived, along with some practical estimators of shear wave speed. These results open up the possibility of faster elastography imaging in deeper tissues, with high accuracy.

Journal Articles

  1. Angular integration autocorrelation approach for shear wave speed estimation in the framework of reverberant shear wave elastography
    H. Asemani, G. Flores, J. P. Rolland, and K. J. Parker
    Proceedings, IEEE UFFC Latin America Ultrasonics Symposium, doi: 10.1109/LAUS60931.2024.10553108 , pp. 1 -3  (2024). View PDF
  2. Angular integral autocorrelation for speed estimation in shear-wave elastography
    H. Asemani, I. E. Kabir, J. Ormachea, M. M. Doyley, J. P. Rolland, and K. J. Parker
    Acoustics, vol. 6, no. 2 , pp. 413 -438  (2024). View PDF
  3. Reverberant magnetic resonance elastographic imaging using a single mechanical driver
    I. E. Kabir, D. Caban-Rivera, J. Ormachea, K. J. Parker, C. L. Johnson, and M. M. Doyley
    Phys Med Biol 68(5) , pp. 055015-1 -055015-16  (2023). View PDF
  4. Theory of sleep-wake cycles affecting brain elastography
    G. R. Ge, W. Song, M. Nedergaard, J. P. Rolland, and K. J. Parker
    Phys Med Biol, vol. 67, no. 22 , pp. 225013-1 -225013-16  (2022). View PDF
  5. Characterization of viscoelastic media using reverberant shear wave autocorrelation estimator
    S. S. Poul, J. Ormachea, and K. J. Parker
    Proceedings, SPIE BiOS, Medical Imaging 2022: Ultrasonic Imaging and Tomography, vol. 12038 , pp. 1203807-1 -1203807-6  (2022). View PDF
  6. Reverberant shear wave phase gradients for elastography
    J. Ormachea and K. J. Parker
    Phys Med Biol, vol. 66, no. 17 , pp. 175001-1 -175001-12  (2021). View PDF
  7. Reverberant elastography for the elastic characterization of anisotropic tissues
    L. A. Aleman-Castañeda, F. Zvietcovich, and K. J. Parker
    IEEE Journal of Select Topics in Quantum Electronics, vol. 27, no. 4 , pp. 7201312-1 -7201312-12  (2021). View PDF
  8. Experimental study to evaluate the generation of reverberant shear wave fields (R-SWF) in homogeneous media
    G. Flores, J. Ormachea, S. E. Romero, F. Zvietcovich, K. J. Parker, and B. Castaneda
    Proceedings, IEEE Ultrasonics Symposium (IUS) , pp. 1 -4  (2020). View PDF
  9. Reverberant 3D optical coherence elastography maps the elasticity of individual corneal layers
    F. Zvietcovich, P. Pongchalee, P. Meemon, J. P. Rolland, and K. J. Parker
    Nature Communications, vol. 10, no. 2 , pp. 4895-1 -4895-13  (2019). View PDF
  10. An initial study of complete 2D shear wave dispersion images using a reverberant shear wave field
    J. Ormachea, K. J. Parker, and R. G. Barr
    Phys Med Biol, vol. 64, no. 14 , pp. 145009-1 -145009-12  (2019). View PDF
  11. Shear wave speed estimation using reverberant shear wave fields: implementation and feasibility studies
    J. Ormachea, B. Castaneda, and K. J. Parker
    Ultrasound Med Biol, vol. 44, no. 5 , pp. 963 -977  (2018). View PDF
  12. Reverberant shear wave fields and estimation of tissue properties
    K. J. Parker, J. Ormachea, F. Zvietcovich, and B. Castaneda
    Physics in Medicine and Biology, vol. 62, no. 3 , pp. 1046 -1061  (2017). View Online